Symmetry for the low-energy effective model of Weyl semimetal

Question

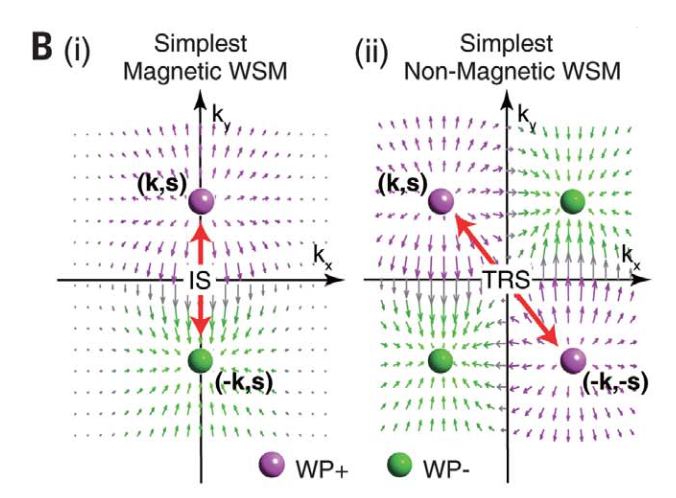
The low-energy effective model for Weyl semimetals (WSM) at a single Weyl point can be written as:

$$H_{w}=\chi \vec{k} \cdot \vec{\sigma}, \tag{1} $$

where $\chi$ is the chirality index, $\vec{k}$ is the crystal momentum, and $\vec{\sigma}$ is the vector of Pauli matrices. The symmetry behavior for $\vec{k}$ and $\vec{\sigma}$ under time-reversal ($\mathcal{T}$) and inversion ($\mathcal{P}$) operations is well-known:

• $\mathcal{T}\vec{\sigma}=-\vec{\sigma}; \mathcal{T}\vec{k}=-\vec{k}$;
• $\mathcal{P}\vec{\sigma}=+\vec{\sigma}; \mathcal{P}\vec{k}=-\vec{k}$;

On the other hand, the Weyl semimetal can be classified as nonmagnetic and magnetic ones. Consider the first one, the system respects $\mathcal{T}$ but breaks $\mathcal{P}$ (we assume there is a total of four Weyl points), we can conclude that: $$\mathcal{T}\chi=\chi \tag{2}$$ For the second case, the system respects $\mathcal{P}$ but breaks $\mathcal{T}$ (we assume there is a total of two Weyl points), we can conclude that: $$\mathcal{P}\chi=-\chi \tag{3}$$

Eq.(2) and Eq.(3) are correct? If not, how Eq.(1) can be used to describe the magnetic and nonmagnetic WSMs simultaneously? Or how does the low-energy model by considering all possible Weyl points respect the original symmetry that the system holds?

Answer 1

I find the answer just by reading this paper:https://www.science.org/doi/epdf/10.1126/science.aav2873.

For the simplest magnetic WSM, we just have two Weyl points, which are related by inversion symmetry, namely: $$\mathcal{P}\chi=-\chi.$$

For the simplest nonmagnetic WSM, we have four Weyl points, in which we have: $$\mathcal{T} \chi = \chi .$$ Note that the different chirality $\chi$ has been represented by different colors in the screenshot.

For each Weyl point, it can be described by $\vec{k}\cdot\vec{\sigma}$, but we need to consider their copies due to the summation of $\chi$ should be zero when we talk about the symmetry, as ProfM told.