When are atomic-orbital-basis (rather than plane-wave) methods appropriate in periodic DFT?

Question

For example, GPAW supports both plane-wave and atomic-orbital basis methods. I know that atomic-orbital basis methods can have difficulty with electrons occupying vacancies for example, but what types of systems are good or bad for atomic-orbital-based methods.

To be clear I would like to know what types of systems have which pros and cons of both methods. I am particularly curious about metal vs semiconductor and surface vs bulk vs nanoparticle etc.

Answer 1

The clearest example in my mind is if you want to understand the orbital-based contributions to some phenomena (e.g. bonding, a reaction energy), particularly if the periodic material being modeled is more like a molecular solid where the chemical picture of orbitals is more intuitive than bands. There are several schemes out there that try to go from PAW to localized basis set-like results though, including the periodic extension to natural bonding orbitals (NBOs), Solid State Adaptive Natural Density Partitioning (SSAdNDP), and the LOBSTER code.

Answer 2

The question is related essentially to solve the Kohn-Sham equation with an atomic-like basis set. In fact, different implementations of DFT are distinguished mainly by their basis set and how they orthogonalize themselves to the core levels. In particular, the choice of basis set forms the core of any electronic structure method.

Dedepending on the choice of basis set and how to orthogonalize, four methods are proposed as shown in the following figure (Nuclei are shown as dots):

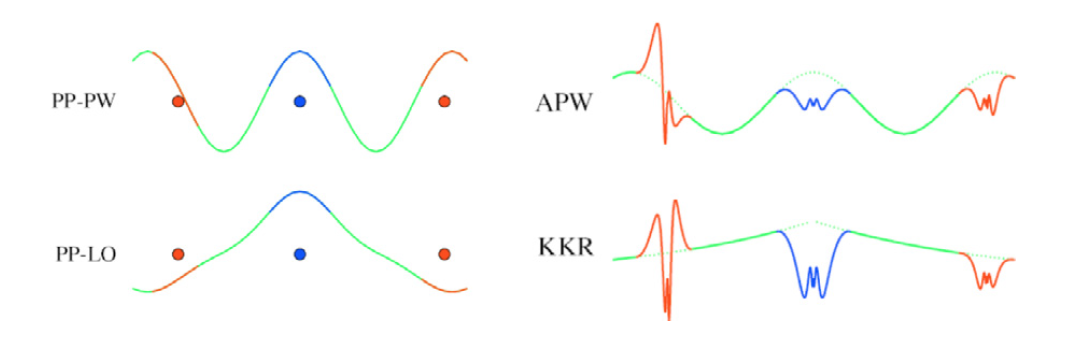
• The all-electron methods APW and KKR on the right substitute (augment) the envelope function (green) with numerical solutions of partial waves inside augmentation spheres (blue and red). Parts inside augmentation spheres are called ‘‘partial waves’’.
• The two figures on the left use a pseudopotential allowing their envelope functions to be smooth, with no augmentation needed. A pseudopotential’s radius corresponds to a characteristic augmentation radius.
• The top two figures use plane waves for envelope functions; the bottom two use atom-centered local basis sets.

The example implementation for these methods:

• PP-PW: VASP
• APW: Wien2K
• PP-LO: Gaussian
• KKR: Questaal (This is what you are looking for!)

For a more detailed comparison between these methods, you can take a look at the implementation paper of Questaal.

When are atomic-orbital-basis (rather than plane-wave) methods appropriate in periodic DFT?

If you want to combine NEGF with DFT to calculate the transport properties of the device, you should use atomic-orbital-basis, as Questaal adopted.