When you see charge densities plotted in the literature, they are usually plotting particular isosurfaces (contours) and are trying to show "where the electrons are". Charge density differences can be useful in trying to understand the changes in a system, for example charge transfer as a molecule approaches a surface (although this often isn't very accurate with common XC functionals). Plotting spin-densities can be very useful to see where the regions of magnetism are, and what nature of magnetism is present (e.g. ferromagnetic or antiferromagnetic).
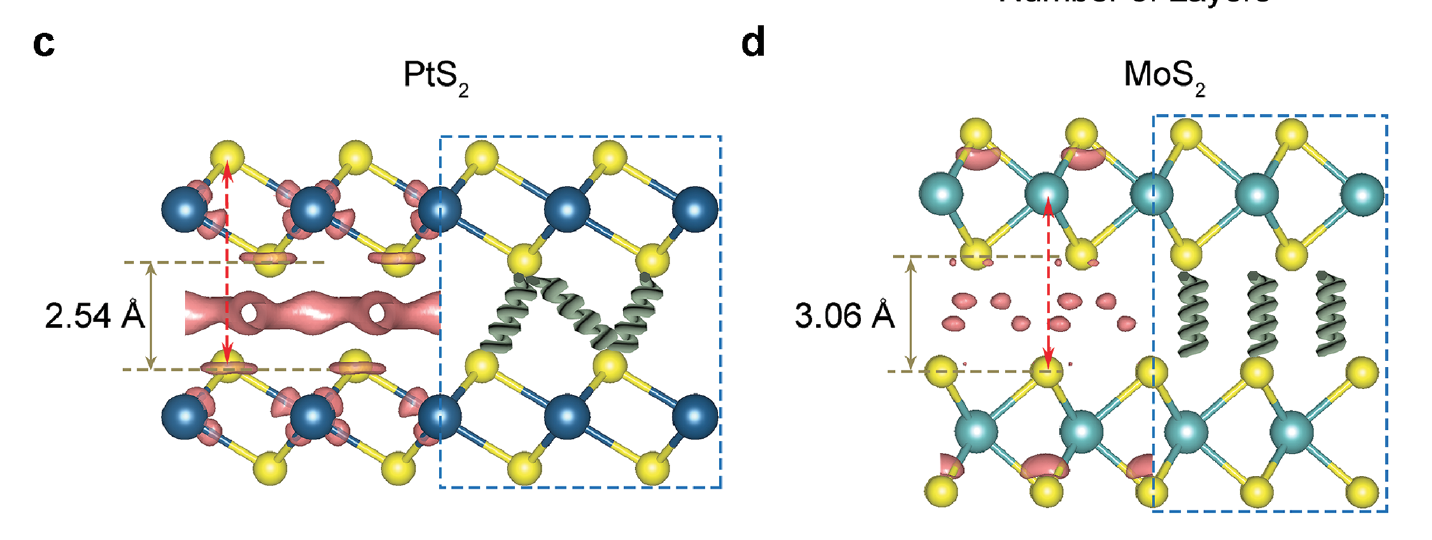
People often try to use the electron density as a proxy quantity for other properties. For example, if you think there should be a covalent bond between layers, then you would expect to find significant electron density in the inter-layer region. If indeed there is a high electron density in this region, then you might argue that this is evidence of a strong bond. In my opinion this is not a very strong argument, because it assumes local interaction with the density, whereas layers could be interacting strongly via non-local interactions (Coulomb interactions such as in ionic materials, for example, or van der Waals interactions) in which case there is no need for a high electron density in the inter-layer region. In fact it is trivial to compute the inter-layer force constant and essentially "measure" the strength of the interaction, so I would always prefer that.
Note that, in principle, the ground state density tells you everything about the ground state of the system -- this is essentially the 1st Hohenberg-Kohn theorem. However, in practice this is not as useful as it may appear at first, since we don't actually know how most of the properties depend on the density, just that they do!