12
$\begingroup$

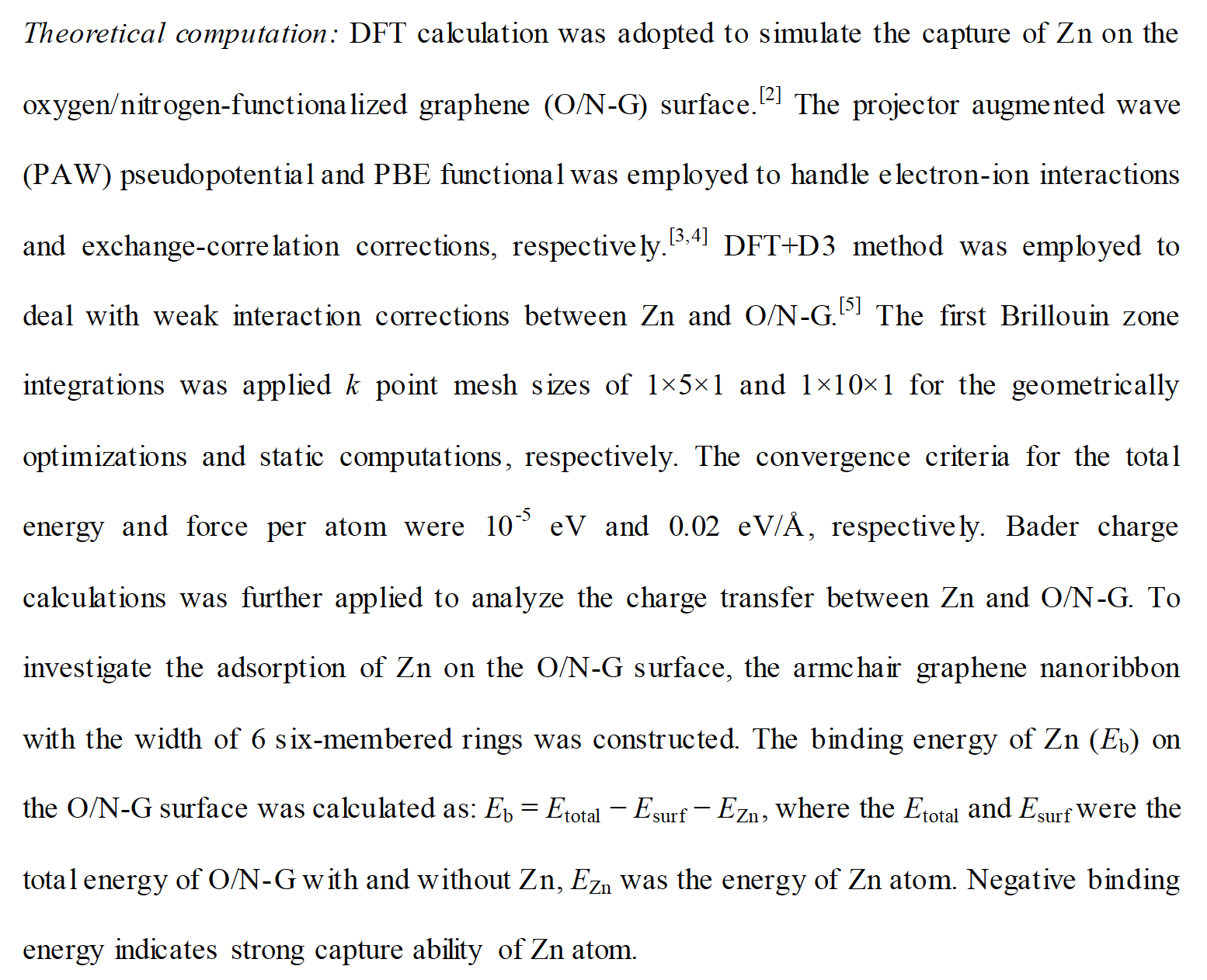
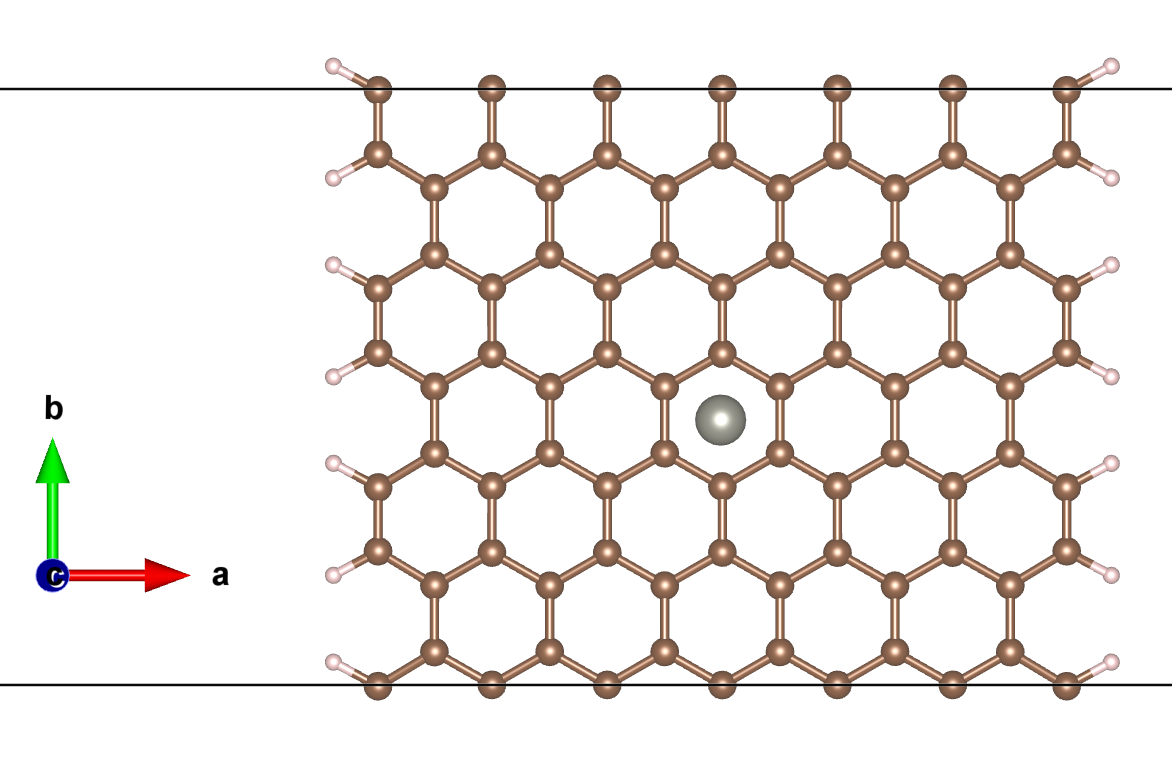
I am trying to repeat the result of this paper for Zn absorbed on the armchair graphene slab.

The relaxed top and side structures are the following:

enter image description here

enter image description here

The relaxed structure is almost the same as the cited paper. Then the energy calculation based on this relaxed structure is listed below:

  • E(slab+Zn)=-759.31696 (eV)
  • E(slab)=-759.30724 (eV)

The energy of Zn is obtained from its bulk calculation:

  • E(Zn)=-1.1078938 (eV)

Then the binding energy is estimated as:

  • $\Delta E$=E(slab+Zn)-E(slab)-E(Zn)=1.0981738 (eV)

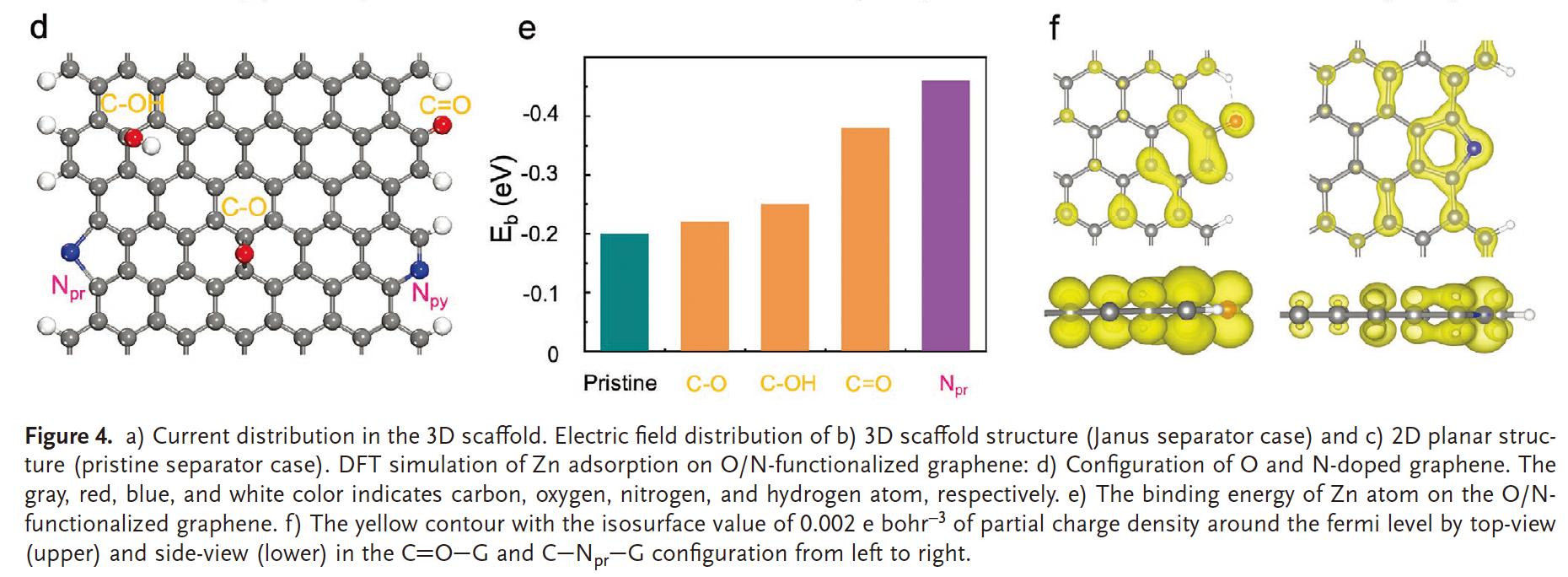
However, the result of the cited paper is -0.2 eV: enter image description here

What am I missing?

For completeness, I will give my input cards for relaxation and static calculations as follows:

 System=2D
 ISTART=0        !startjob: 0-new 1-cont 2-samecut
 ICHARG=2        !charge: 1-file 2-atom 10-const
 ENCUT=500       !energy cutoff in eV
 EDIFF=1E-6      !stopping-criterion for electronic upd.
 NELM=300        !nr. of electronic steps
 ISMEAR=0        !part. occupancies
 SIGMA=0.05      !broadening in eV -4-tet -1-fermi 0-gaus
 IALGO=38
 LREAL=Auto
 IVDW=11

 #------------------
 #ISPIN=2        !spin polarized calculation (2-yes 1-no)
 #MAGMOM=128*0   !initial mag moment / atom
 #LSORBIT=.TRUE. !if .TRUE. switches on spin-orbit coupling
 #SAXIS= 0 0 1   !quantisation axis for spin
 #ISYM=0         !symmetry: 0-nonsym 1-usesym

 Dynamic:
 ISIF=2          
 IBRION=2
 NSW=300
 EDIFFG=-0.005

 Parallelization:
 NPAR=8
 #KPAR=4

 Output:
 LCHARG=.FALSE.
 LWAVE=.FALSE.

=========================================

 System=2D
 ISTART=0        !startjob: 0-new 1-cont 2-samecut
 ICHARG=2        !charge: 1-file 2-atom 10-const
 ENCUT=500       !energy cutoff in eV
 EDIFF=1E-6      !stopping-criterion for electronic upd.
 NELM=300        !nr. of electronic steps
 ISMEAR=0        !part. occupancies
 SIGMA=0.05      !broadening in eV -4-tet -1-fermi 0-gaus
 IALGO=38
 LREAL=Auto
 IVDW=11

 #------------------
 #ISPIN=2        !spin polarized calculation (2-yes 1-no)
 #MAGMOM=128*0   !initial mag moment / atom
 #LSORBIT=.TRUE. !if .TRUE. switches on spin-orbit coupling
 #SAXIS= 0 0 1   !quantisation axis for spin
 #ISYM=0         !symmetry: 0-nonsym 1-usesym

 Dynamic:
 #ISIF=2          
 #IBRION=2
 #NSW=300
 #EDIFFG=-0.005

 #Parallelization:
 NPAR=8
 #KPAR=4

 Output:
 #LCHARG=.FALSE.
 #LWAVE=.FALSE.

For convenience, the computational details for the cited paper are put below: enter image description here

Improve this question
$\endgroup$
7
  • 2
    $\begingroup$ Can you share your INCAR? Are you reproducing their exact theory? $\endgroup$
    – Tristan Maxson
    Commented Oct 13, 2020 at 16:03
  • $\begingroup$ The energy of Zn was obtained from bulk but is not the bulk energy right? $\endgroup$
    – Camps ♦
    Commented Oct 13, 2020 at 18:03
  • 1
    $\begingroup$ @Camps I calculated from bulk because from this information: [The energy of the Zn atom was calculated using the metallic Zn configuration]. Am I wrong? How can estimate the energy of the single Zn atom? $\endgroup$
    – Jack
    Commented Oct 13, 2020 at 21:16
  • 1
    $\begingroup$ Yes, you should use the energy of a single atom, as it is a single atom interacting with your slab. $\endgroup$
    – Camps ♦
    Commented Oct 14, 2020 at 12:26
  • 1
    $\begingroup$ Using the energy of a single atom can mean many things. It should be a single atom in its standard state (Zn metal) not a single atom in gas phase. Technically you could use a single atom in gas phase as the reference if you wanted to calculate a true "binding" energy but this sort of binding is unreasonable to look at. Using the metallic state you would get a formation energy. Maybe this is the confusion. $\endgroup$
    – Tristan Maxson
    Commented Oct 14, 2020 at 15:06

1 Answer 1

Reset to default
7
$\begingroup$

As mentioned by @Camps in the comments, the Zn reference used in the paper is almost certainly that of an isolated Zn atom in the gas phase. If I understand correctly from briefly scanning the paper, the source of Zn in the device (as viewed from the graphene-coated separator) will be Zn2+ ions dissolved in the electrolyte - not quite the same thing as Zn in the gas phase, but certainly not Zn bulk.

In order to get an accurate result, take the cell you used for the slab+Zn calculation, delete the slab and recompute the total energy.

I agree that the description "metallic Zn configuration" in conjunction with individual Zn atoms is somewhat confusing; it may have been intended to indicate the (neutral) charge state or a specific magnetic configuration - I'm sure the authors would be happy to clarify!

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .