OF-DFT: Orbital-free density functional theory
Hohenberg and Kohn established that the ground state energy, $E$, of interacting electrons in a potential, $v(\mathbf{r})$, is a functional of the electron density, $n(\mathbf{r})$:
$$
\tag{1}
E[n] = F[n] + \int \mathrm{d}\mathbf{r} \, v(\mathbf{r}) n(\mathbf{r}) .
$$
While this statement is formally true, we do yet not have a convenient way to compute the "universal functional" $F[n]$ exactly for most cases. To carry out OF-DFT, one chooses an explicit form for $F[n]$, likely an approximation, and varies the electron density to find the particular $n(\mathbf{r})$ that minimizes $E[n]$, yielding the ground state. Quantities like forces and stresses then follow from Hellmann–Feynman-type formulas.
Both the advantages and challenges of OF-DFT stem from its simplicity; wave functions and density matrices are eschewed altogether. For cases when OF-DFT is suitably accurate, it is extremely attractive from a computational standpoint, in significant part because $n(\mathbf{r})$, the sole working variable, grows only linearly with system size. However, for much of the periodic table, OF-DFT remains less accurate than other electronic structure methods.
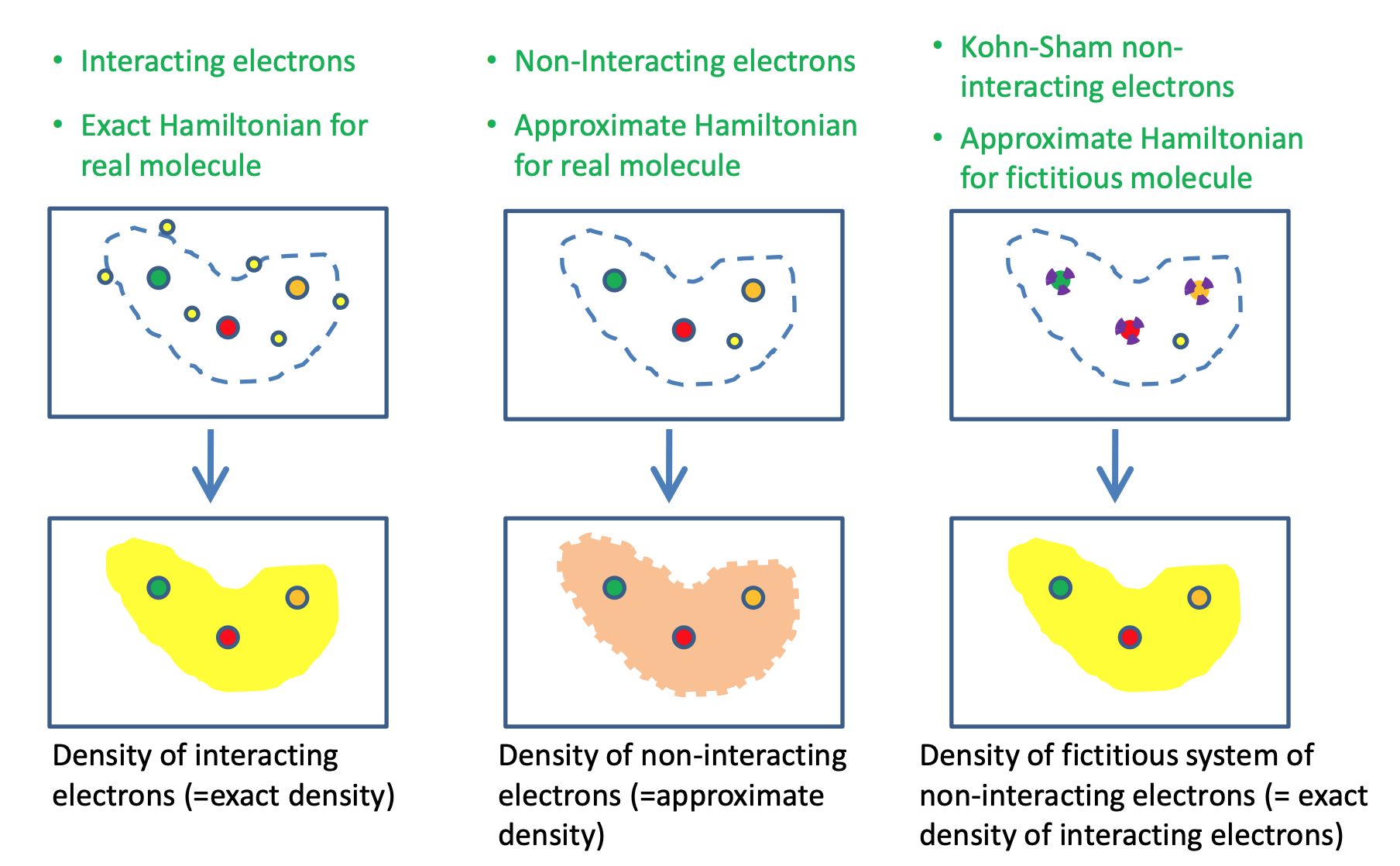
Modern implementations of OF-DFT often build on the insights of Kohn and Sham, who considered $F[n]$ in the form
$$
\tag{2}
F[n] = T_s[n] + E_{Hxc}[n] ,
$$
where $T_s[n]$ is the kinetic energy of an auxiliary system of noninteracting electrons (with the same electron density as the interacting system) and $E_{Hxc}[n]$ subsumes electrostatic, exchange, and correlation contributions. The full Kohn-Sham scheme determines $T_s[n]$ implicitly, but exactly, following the introduction of single-electron orbitals. The corresponding orbital-free approach, in contrast, approximates $T_s[n]$ with an explicit density functional, while utilizing the same approximations for $E_{Hxc}[n]$. Simple approximations to $T_s[n]$ include the Thomas-Fermi functional,
$$
\tag{3}
T_{TF}[n] = \frac{3}{10}(3\pi^2)^{2/3}\int \mathrm{d}\mathbf{r} \, n^{5/3}(\mathbf{r}) ,
$$
and the Weizsäcker functional,
\begin{align}
T_W[n]
& = -\frac{1}{2} \int \mathrm{d}\mathbf{r} \, n^{1/2}(\mathbf{r}) \nabla^2 n^{1/2}(\mathbf{r}) \tag{4}\\
& = \int \mathrm{d}\mathbf{r} \, \left[ \frac{1}{8} \frac{|\nabla n(\mathbf{r})|^2}{n(\mathbf{r})} - \frac{1}{4} \nabla^2 n(\mathbf{r}) \right],\tag{5}
\end{align}
both of which are exact for certain limiting cases and predate the Hohenberg-Kohn theorems by decades.
For more (disclaimer: from my perspective), here is a recent review of successful OF-DFT applications in materials science:
- W.C. Witt, B.G. del Rio, J.M. Dieterich, and E.A. Carter, Orbital-free density functional theory for materials research, Journal of Materials Research 33 (2018) (DOI: 10.1557/jmr.2017.462).