Can we "invert" Density Functional Theory through sufficiently accurate experiment?

Question

Cross-posted on Physics.SE.

---

The famous Hohenberg-Kohn theorems say that there is a one-to-one mapping between the many-body Hamiltonian, $\mathcal{H}$, of a solid and its ground-state electron density $\rho(\mathbf{r})$. As far as I understand, this also means that all the properties of the ground-state wavefunction are encoded in the electron density itself (though perhaps not in a simple way).

Density functional theory aims to solve for this ground-state electron density $\rho(\mathbf{r})$ through various simplifications and manipulations of $\mathcal{H}$ to make the process computationally tractable.

I am interested in the reverse process, where an experimentalist comes up to me with their measured $\rho(\mathbf{r})$. In principle, a sufficiently accurate measurement of the electron density can be done with X-ray scattering (or electron microscopy) to obtain $\rho(\mathbf{r})$. Typically, such measurements of $\rho(\mathbf{r})$ are only used to get the positions of the atoms in a crystal, but the Hohenberg-Kohn theorems and DFT suggest you could do a lot more with $\rho(\mathbf{r})$.

So my question is: Given an experimentally determined $\rho(\mathbf{r})$ to arbitrary accuracy, what can we say about a material's properties using "inverse" DFT?

As a followup, what experimental accuracy for $\rho(\mathbf{r})$ is needed to accurately determine those material properties?

Answer 1

"Inversion" is common in wavefunction-based quantum mechanics, for example the RKR inversion method which constructs a potential energy function based on information that can be obtained from spectroscopic experiments, such that a Hamiltonian using this potential, when fed through the Schroedinger equation, will give eigenvalue differences that match the experimentally observed spectra. Likewise similar things can be done if the experimental data doesn't only contain energies (eigenvalues) but contains wavefunction information (such as Franck-Condon factors or spectroscopic intensities). The RKR method has a lot of historical significance, and it still sometimes used today, including (sometimes) as a stepping stone towards "Direct Potential Fitting" which is the state-of-the-art method for obtaining an empirical potential function for a small molecule from experimental spectroscopic data.

A similar thing could be done if we had an "experimental" ground-state electron density, because:

HK Theorem 1: The external potential, is a unique functional of the electron density

which means $V(\mathbf{r}_i) = V[n(\mathbf{r})]$, which can then be plugged into the canonical expression for the many-electron time-dependent Hamiltonian:

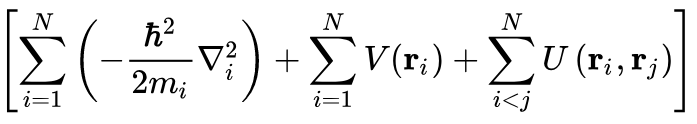
Then since the kinetic energy (the first sum) and the electron-electron interaction energy (third sum) are "universal operators", meaning that they're the same for any $N$-electron system, plugging in our obtained $V(\mathbf{r}_i)$ gives us a completely characterized Hamiltonian, which means all properties of the system, including the many-body wavefunction follow, though if we had the density we could have just got those properties properties from:

$$\left\langle \hat{O} \right\rangle = \textrm{Tr} \left(\hat{\rho}\hat{O} \right), ~ \hat{\rho} = n(\mathbf{r}). \tag{2}$$

There's some caveats:

• Just as in the "Direct Potential Fitting (DPF)" using wavefunction-based quantum mechanics where you need a "model" for the potential energy function, if you don't know the functional $V[n(\mathbf{r})]$ you would need to use a model functional and the accuracy of the $V(\mathbf{r})$ that you get, will depend on how good your model functional is.

• Obtaining the Hamiltonian can be considered obtaining the "material's properties" (to use your words), but by "material properties" you might mean things like "conductivity". In the DPF case we do obtain things like bond lengths from the fitting procedure, but only because bond length is a property defined based on an easily accessible property of the potential energy function which was obtained in the DPF process: likewise any properties of the material which are defined based on easily accessible properties of $V(\mathbf{r})$, will be obtained, but other properties which are defined differently, could be better obtained from Eq. 2.

Finally to answer your follow-up question:

"As a followup, what experimental accuracy for ρ(r) is needed to accurately determine those material properties?"

You can use error propagation techniques to determine what an error in $n(\mathbf{r})$ will imply for the errors of $\left\langle \hat{O} \right\rangle$ from Eq. 2, but for obtaining properties based on $V(\mathbf{r})$ this paper by Bob Le Roy would be a good place to start, but beware of the fact that there will be a contribution to the final uncertainty in the material property coming from the choice of the model (functional in your case, potential energy functioni n the Schroedinger-based DPF case) you use, which can be extremely difficult even to define, and is unfortunately ignored usually in research papers even by experts.

Answer 2

This is not a direct answer to your question, but I hope to get the ball rolling.

In the question, you ask about reverse-engineering an experimentally obtained density to say something about density functional theory (DFT). While I am not aware of any examples of this, there are examples of reverse-engineering a computationally obtained density to say something about density functional theory.

As an example, consider this paper. They introduce "a self consistent algorithm for reverse engineering the exact potential from the known time-dependent charge and current densities". What does this mean? They solve a simple problem in a numerically exact way to obtain the charge (and current) density, and then reverse-engineer them to obtain the exact Kohn-Sham potential. In this particular example, they are interested in time-dependent DFT, and their work shows some interesting features of the corresponding Kohn-Sham potential, such as a non-local dependence of the charge density in both space and time.

Overall, while this is not a direct answer to your question about "experimentally determined densities", I hope it helps see what can be accomplished by reverse-engineering DFT.